The Intrinsic Production Rate

The ionizing photon production efficiency, \(\xi_{\rm ion}\), is one of the main quantities we need to calculate for the simulated galaxies (see this post).

The ionizing photon production efficiency

As outlined in Naidu et. al 2020, \(\xi_{\rm ion}\) can be calculated directly from the SED:

\[\xi_{\rm ion} = \frac{N(H^0)}{L_{1500}} [\rm Hz/erg ]\]

This is done by:

integrating the flux produced below the Lyman limit (912 Angstroms) to get \(N(H^0)\) [photons/s/Hz]
normalizing by the UV Luminosity/SED-flux at 1500 Angstroms [ergs/s]

My calculation

Given a rest-frame SED \(f_{\nu}\) in units of [luminosity/frequency] (FSPS by default does not include the distance; with a distance modulus this can be converted to the usual units \(ergs/Hz/cm^2/s\)) as a function of wavelength, \(\lambda\), I calculated:

\[N(H^0) = \int_{\nu_{912}}^{\nu_{0}} \dfrac{f_\nu \nu}{h \nu} {\rm d} \nu = \int_{0}^{912} \dfrac{f_\nu \nu}{h \lambda} {\rm d} \lambda\]

and

\[L_{1500} = \dfrac{\int_{1450}^{1550} f_\lambda {\rm d} \lambda}{100}\]

Results

I took the DREaM catalog cut with \(M_{\rm gal}>10^{10} M_{\odot}\) (to make it more manageable for testing), and calculated \(\xi_{\rm ion}\) from each galaxy, as outlined above.

Here is the distribution I expect (from Fig 2 of Naidu et al. 2020):

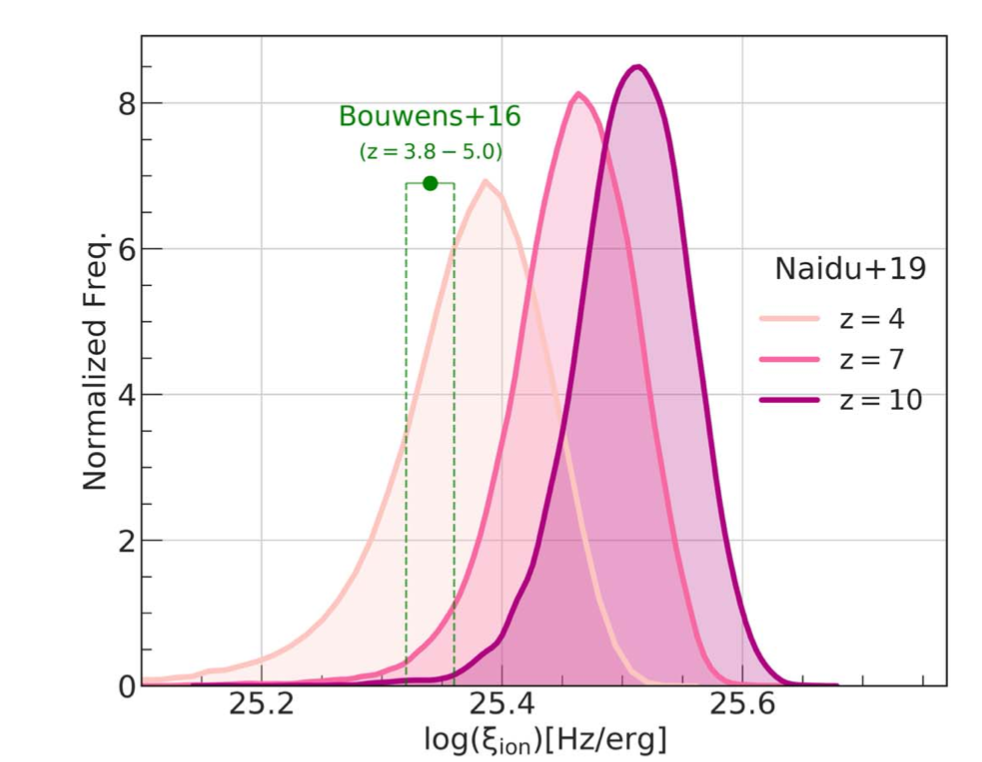
Here is the distribution of \(\xi_{\rm ion}\) I get:

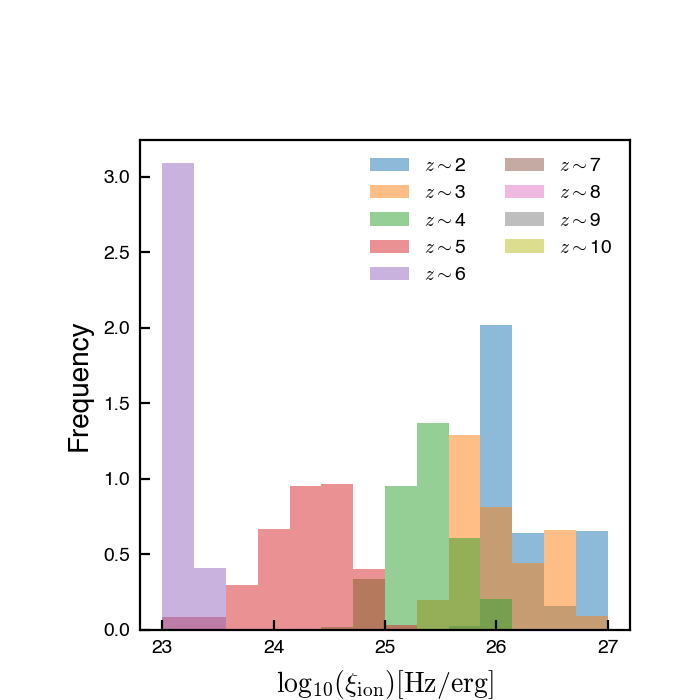
I truncated the plot at \(\xi_{\rm ion}=23\), to see the results a bit better. This means I am not showing the higher redshift data (z>6). My calculation agrees with the Naidu model, and the Bouwens data around redshift 4, but I get a lot more variation. I’m not sure whether this is because I made a mistake in the calculation or because of modelling differences.

Next steps

Triple check my \(\xi_{\rm ion}\) calculation
Check the literature to see if mine or Naidu’s results are more consistent with observations
Look to see what SED modelling assumptions will most strongly affect \(\xi_{\rm ion}\)

« Galaxy Surveys for EoR

The Escape Fraction »